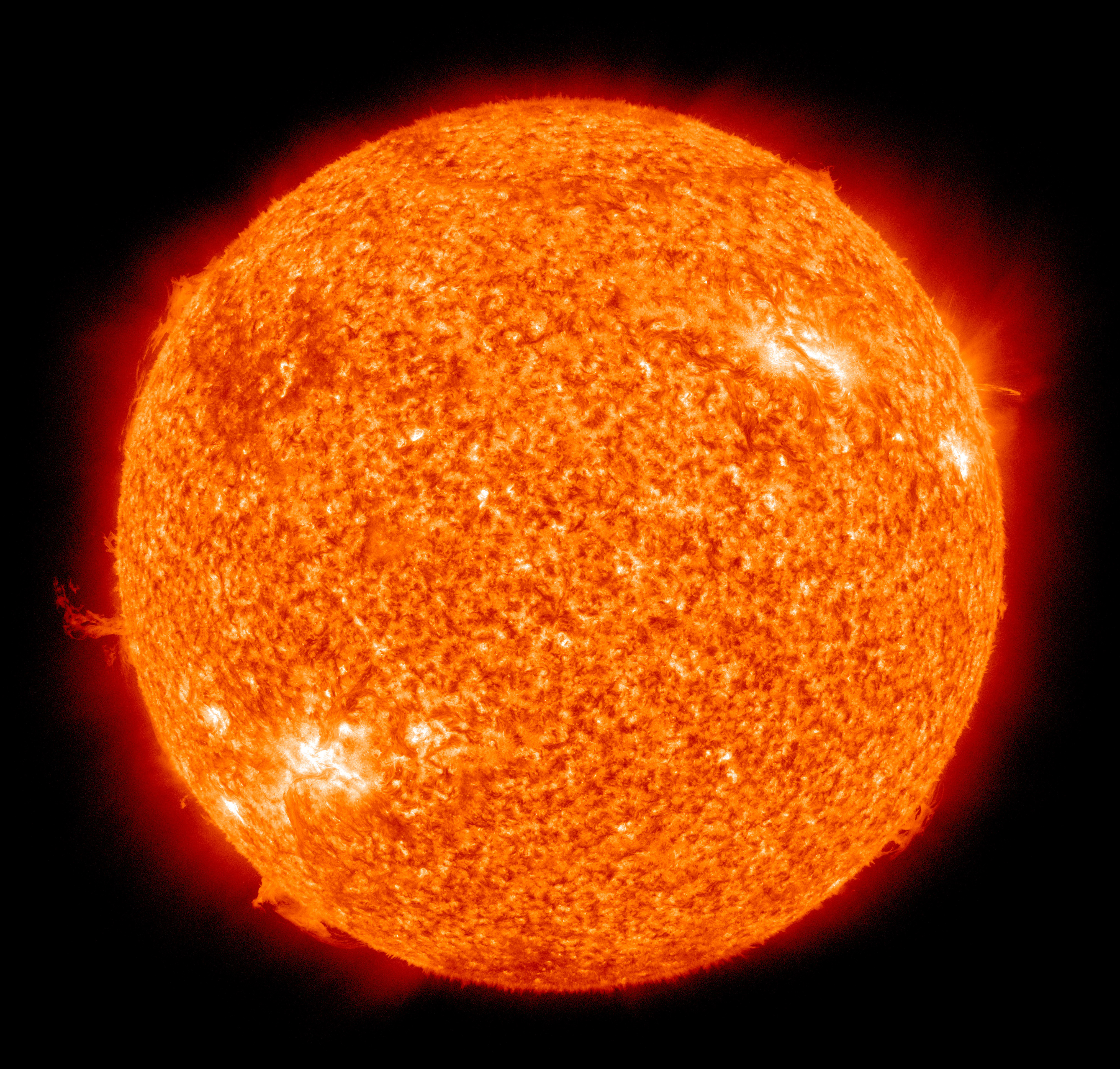
Why is the sun so powerful? Why do nuclear weapons create such a big boom? The answer can be broken down to mass-energy equivalence, as expressed by the most famous equation in all of science.
Contrary to popular belief Einstein was not the first one to ever propose a relationship between mass and energy, but he is the one that created the formula, which is a natural result from his theories of relativity.
An examination of the wonderfully simple equation reveals the answer as to why atomic physics deals with such powerful levels of energy.
"c" stands for the speed of light- 299,792,458 meters per second. The equation then squares this number and multiplies that by the mass of the object. There is your answer in joules. As you can already guess, the number of joules (and thus the amount of energy measured) is staggering. Put simply, it's the most efficient release of energy known to science. Whereas the breaking of chemical bonds releases some energy, it pales in comparison to any nuclear process where a mass-energy dynamic is present. Let's try an example:
Say that one kilogram of matter is converted entirely into energy.
1 x 299,792,458^2 = 89875517873681764 joules.
That is equivalent to 21.480 megatons of TNT- a yield nearing one of the most powerful thermonuclear devices ever tested.
 |
| The Castle Yankee device, which converted less than one kilogram |
And that's only one kilogram, one!
Mass-energy equivalence is serious stuff, friends.
What makes it all go is the loss of mass in a system. This lost mass then gets converted entirely into energy. When a nuclear weapon goes boom, the spectacularly destructive results that you see is due to a certain loss of mass in the original system. The same forces are at play inside the cores of the stars.
The sun for instance, loses 4 million metric tons per second, converting some of that lost mass into energy. The slightly less massive sun then radiates that energy at about 3.846 x 10^26 watts per second, or around 91.921 petatons of TNT per second (that's 91.921 quadrillion tons), over a trillion times more powerful than the Tsar Bomba. More massive stars do this at even higher rates and produce even more energy.
That's mass-energy equivalence in a nutshell.

No comments:
Post a Comment